Transition of Maths Teaching and Learning from Primary School to Secondary School

Saturday 13 Jun 2020

Recently our G6 students finished their study of proportionality. As the last topic of national curriculum of G6 Mathematics, it is also drawing towards the end of the Mathematics study in primary level. In fact, the study of the chapters in this whole term is not only the summary and extension of the knowledge and skills we have practiced throughout the whole primary level, but also the paving and laying out the foundation for the next stage of mathematics learning.From our previous experience, there were always some students who found Maths became more challenging when they transited to G7. Especially when some entered to term 2 of G7, they found themselves lost in Maths lessons. “When my teacher explaining factorization to us, I could understand very clearly at the beginning. But from some step, I had no more idea what the teacher was talking about. When I was practicing some of the questions myself, I do not even know how to start.”

Today we are going to take the topic of proportionality for example, to have a discussion on the different sets of knowledge and Mathematical skills required from primary school to middle school, which will be mainly in the following aspects:

1. Calculation: from arithmetic to algebra – from numerical calculation to manipulation of algebraic expressions;

2. Problem solving: changes of perspectives from numerical analysis to variable relations.

Both of the two areas of main changes happened in the topic of proportionality. Now let’s take a look at one set of questions we practiced during our topical reviews. Students need to tell what type of proportion between the two variables in each case: direct proportion, inverse proportion or no proportion:

1. The perimeter of rectangle fixed, length and breath.

2. The first term of ratio fixed, the second term and the value of ratio.

3. Circumference of a circle and its radius.

4. The total number of product fixed, the number of qualified products and the passing rate.

5. The mass of a coffee bean bag fixed, the number of bags and the total mass.

6. The volume of a cone fixed, its base area and its height.

Despite of the limitation of this type of questions, with no numerical calculation needed, it still requires a relatively high level of Maths thinking and skills. Take Question 3 for example, students not only need to know the formula of area of a circle, they should also be able to perform basic algebraic manipulation purposefully to obtain S⁄r=πr, and then the capable ones could tell the two quantities are not directly or inversely proportional as certain conditions not satisfied. And these six questions cover knowledge from G2 to G6: rectangle, product passing rate, price, ratio, circle and cone… with more advanced and algebraic requirement.

Whether students could show competence in G6 Maths, and further success in the next stage of study, it depends on whether they have a solid foundation in the following three aspects:

1. Numerical calculation. Do it correctly, then fast. With well acquired number sense and the help of a range of operation rules, simplification should also be performed whenever necessary;

2. Understanding of various quantitative relations. The teaching and learning of Maths is a process of spiraling upward. As mentioned in previous example, exercises in the topic of proportionality also required wide range of knowledge and proficiency in many other topics.

3. Information processing capability. It’s also one of the foci and objectives which we would like the students to achieve in the subject. From the known to the unknow, prerequisites to logical conclusion, relations among quantities – in fact most of problems solving in Maths depend on such capability of processing and handling information.

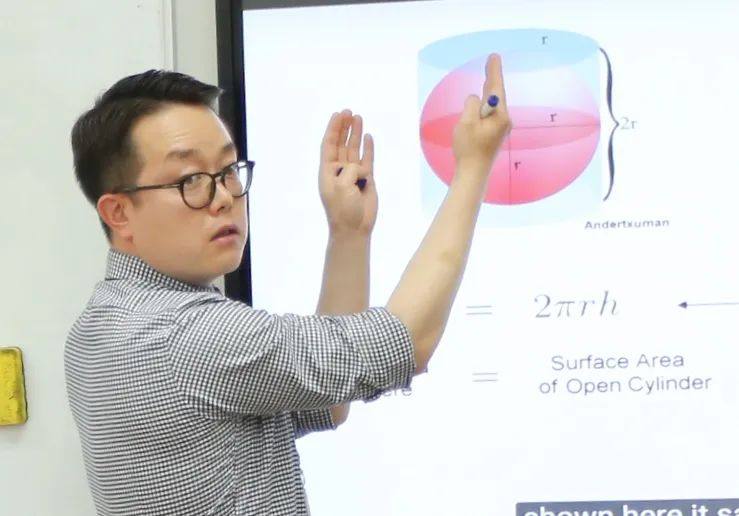
All the above three aspects put forward higher requirements on students’ mathematical ability in the transition period from primary school to middle school. Solid foundation in knowledge and skills, as well as good study habits have always been the cornerstones of academic success in every stage of education.

In RDFZ King’s College School Hangzhou, our Mathematics teaching and learning are concept-oriented, with high requirement on knowledge structure. While in each lesson, we try to embed the concept in a real-life problem, learning from hands on activities as many as possible.

In this topic of proportionality, we did two projects. One was to let students become landscape designers, who not only had to complete the design in given scale, but also communicate details with customer by email. For instance, if one student wanted to lay a gravel path in the garden, he needed to think about the thickness of this gravel path, and the cost of gravel per cubic meter; if one student wanted to plant a cherry tree, then what is the price of a cherry tree with main stem of 8 cm diameter? All these factors needed to be considered.

Another project was integrated with the chapter of cylinder and cone. Students were to make a cylindrical pen bag with specific measures. They need to calculate the circumference of circular base and height with certain scale ratio, sketch on a paper then cut fabric accordingly to sew it into a real pen bag.

What we have discussed in this article are barely our teachers’ own opinions on Maths teaching and learning, which might be limited by our own experiences, group of students or the nature of our education system. Please share with us your insights or experiences on this, or if you have any inquiry, please feel free to contact me at: tom.li@rkcshz.cn.